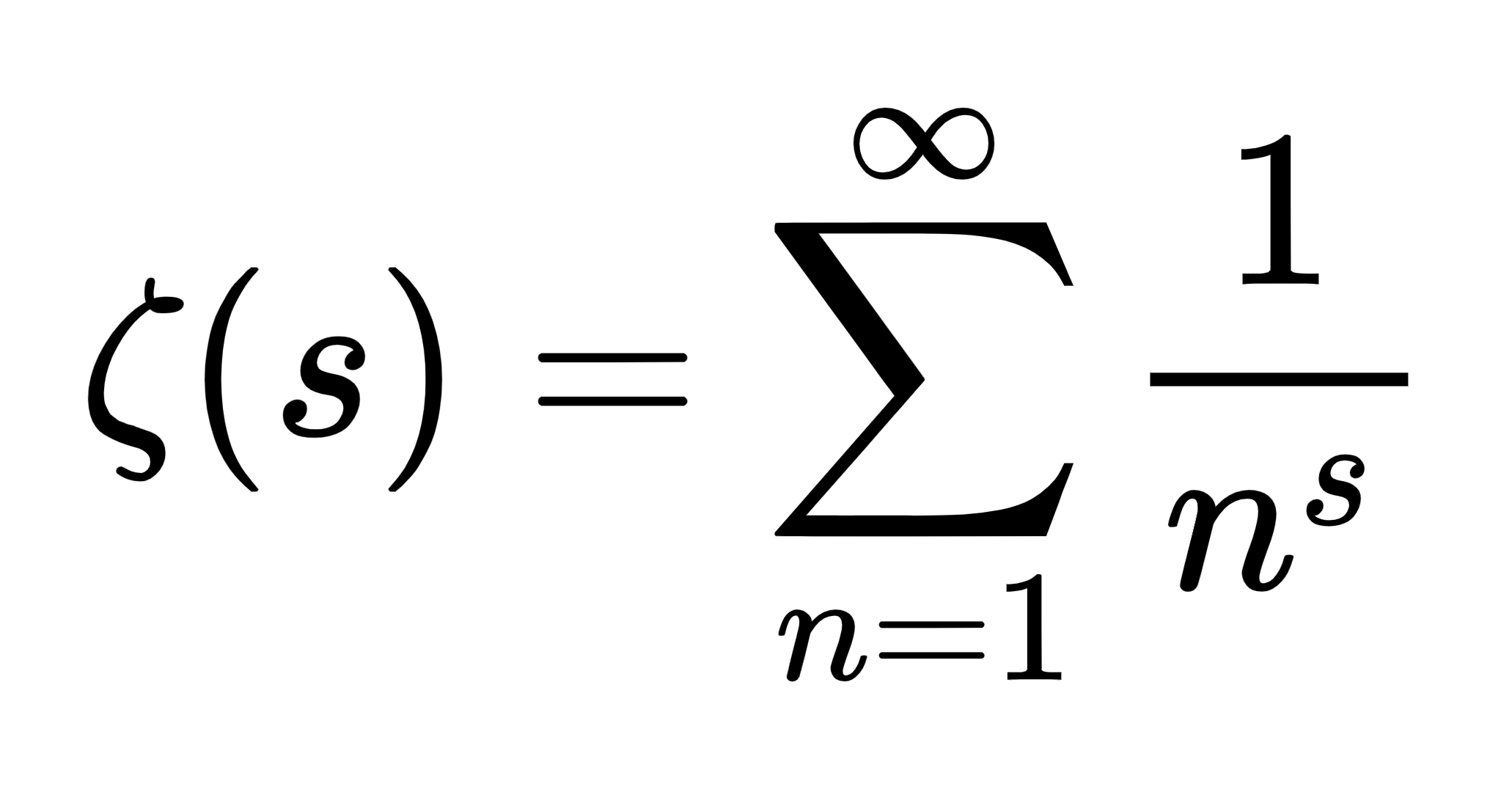
1. Introduction
Back in 1859, German mathematician Bernhard Riemann introduced us to 6 important hypotheses...
Just kidding, did you really think I'd solve the Riemann Hypothesis here? April Fools joke!
Half of the title is correct, though. Today, we shall discuss a problem from the 2016 Maths Olympiad shortlist, proposed by Mihai Piticari. The problem goes as follows: Determine all finite groups which have proper subgroups only of order 2 or 3.
Solution:
First off, let us observe that the cardinal of the required group G is of the form \(2^i3^j\). By the Sylow theorem, we will have a subgroup of order \(2^i\) and a subgroup of order \(3^j\). If both of them are positive and one is bigger than 2, then we will have a subgroup larger than order 2 or 3. Thus, G can only have \(2^k\), \(3^k\) or 6 elements.
In the first case, we get that all elements have order 2, and, thus, \(xy=yx\), for all elements of G. If this happens, we have a subgroup of order 4, made of \(x, y ,xy ,e\), so the only groups working in this case are Klein's group and the group of order 2, C2.
In the second case, we shall use the equation of the classes. Let us observe that, if 2 elements that aren't part of the same subgroup commute, then we shall have a group of order 9. By the equation of the classes, we have that \(3^k=|Z(G)|+Σ\frac{|G|}{|C(G)}\), where the sum is made by a complete system of representatives with non-trivial orbit.. Since \(C(G)<4\) by the observation above, it is easy to see that \(C(G)=3\), and, thus, if k is bigger than 2, we will need the center of G to have a number of elements that is a multiple of 3. Thus, we get that the only possible groups are the group of order 3 and the group of order 9. Thus, G is C3 or C9 or C3xC3.
In the third case, we have that G is either C6 or C3xC3.
This problem might not have been as easy as the Riemann Hypothesis, but it was fun nonetheless. Seriously though, it would be fun if someone actually proposed a correct solution of the actual RH on the first of April and someone disregarded it as a prank. Jokes aside, tune in next time for some stuff about complex numbers, another algebra problem, another Millennium Prize problem, or something completely random!
Cris.